Angling For Gamma-ray Bursts
Student Handout
Introduction
Getting the direction to a gamma-ray burst in the sky is tricky. The direction to a source that emits optical light is relatively easy to find using aluminum or glass mirrors to focus the light. But gamma rays have such a high energy they pass right through glass and aluminum, so a regular mirror is useless. In fact, the original satellites used to discover GRBs could only get a very rough position in the sky.
Modern satellites like Swift will be able to get much more accurate directions to GRBs using sophisticated detectors, but for decades this option was not available. What could astronomers do?
An alternative method was cleverly devised using the timing of a GRB. Many space probes sent out into the solar system are equipped with detectors that are sensitive to gamma rays. The direction cannot be determined from any given probe, but the exact time of the event can be recorded. When a gamma-ray burst explodes billions of light years away, the light rays appear to come in as parallel rays to different detectors at widely spaced locations. This fact, together with the timing of the event from different satellites, can be used to determine the direction to the GRB.
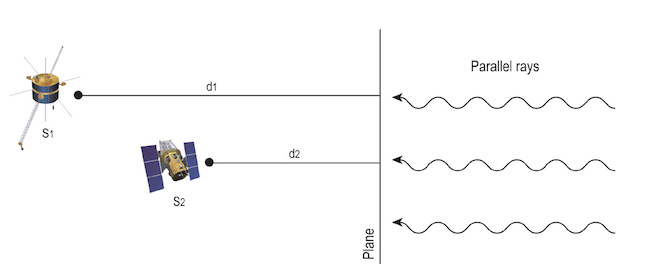
In the figure above, two satellites WIND (S1) and Swift (S2) see the light rays from the gamma-ray burst at different times, since they are at different distances from the burst source. Imagine a plane lying in front of the satellites at some arbitrary distance, perpendicular to the incoming gamma rays. Because the gamma-ray burst is so far away, the gamma rays arrive at the plane at the same time. They then travel different distances d1 and d2to reach the satellites. Light takes a finite time to travel those distances, and the delay between the detection of the burst by the two satellites can be used to determine the direction to the burst.
Note that d1 and d2 are parallel. This is because they see the burst coming from the same direction.
So, if each satellite records the time it receives a burst of gamma rays, then the direction to the GRB can be found knowing the relative positions of the satellites. In fact, since 1990, scientists have used this technique to locate many GRBs on the sky, using what is called the interplanetary network of satellites (IPN). The member satellites change as old ones are shut down and new ones are added, but in general there are about six satellites in the IPN, and they locate about 150 GRBs per year. Swift itself will be a part of the IPN; although it will directly determine the direction to a GRB, it's always nice to have an independent method as backup.
In this activity, you will determine the direction to a burst using the times it is detected by three different spacecraft located somewhere in the solar system. We are going to assume that all the spacecraft are in the plane of the Earth's orbit around the Sun; that is, there is no third dimension. We are only concerned with two dimensions, x and y. We will also assume the burst is billions of light years away, so the incoming gamma rays are traveling along parallel lines.
Step 1: Plotting the Satellites and Calculating the Delay Times
You will use your graph paper to represent the Earth's neighborhood in space. Near or at the center of the graph paper, mark one point as the origin (0,0). This will be the location of the Earth. Now draw and label the x and y axes.
Each square on the graph paper will have a length of one light minute. A light minute is a measure of distance, defined as the distance light travels in a vacuum in one minute, approximately 1.8 x 1010 meters (m).
Question 1: Using a ruler, measure the length of a row of ten squares, then divide by ten to get the length of the side of one square in centimeters (cm). Repeat this procedure using different rows of squares, then calculate the average value. Round numbers to the nearest 0.1 cm.
This number is the scale of the grid in cm/light minutes.
Using the data listed below, plot the locations of the satellites on the grid. Label the satellites "S1" and "S2". Note that Swift is in low Earth orbit, so on the scale of this grid it is essentially at the same place as Earth (at 0,0).
| Satellite Name | Satellite Designation | Coordinates (light minutes) |
| WIND | S1 | (5, 10) |
| Swift | S2 | (0, 0) |
On May 16, 2000 at 9:23:00 UT (Universal time, sometimes called Greenwich time), gamma rays from a GRB passed the plane (see Figure 1). At 9:28:00 UT satellite S1 detected the gamma-ray burst. At 9:36:00 UT satellite S2 detected the same gamma-ray burst.
Question 2: How long did it take (in minutes) for the light to reach each satellite after it passed by the parallel plane?
Question 3: Given that the speed of light is 3 x 108 m/s (be careful to note the units!), what distance did the light travel (in meters) to reach the satellites after it passed the plane?
Question 4: How many light minutes distance did the rays travel after they passed the plane? (Recall that one light minute is about 1.8 x 1010 meters.)
Compare your answers to questions 2 and 4. What do you learn from the comparison?
Step 2: Plotting the Delay Times Using the Light Rulers
You have been provided with T-shaped "light rulers", each of which has a long arrow along the vertical leg, and a shorter arrow along the top crossbar. The arrows are perpendicular, and the long arrow intersects the short one at the middle. These will represent the different distances that light travels from the plane to the two satellites; hence the name "light rulers". The purpose of these next calculations is to find the lengths for the light rulers.
Question 5: Using the grid scale you calculated for Question 1, convert the distances the light traveled in light minutes (Question 2) to centimeters on the graph paper. Round numbers to the nearest 0.1 cm.
Get one of the light rulers. Starting at the tip of the long arrow (where it meets the short one), measure along the long arrow and mark the point on that line such that the length is the length you found in Question 5 for Satellite 1 (see illustration). Label this ruler S1. Repeat this procedure for the other ruler using the data for S2. Don't forget to label the second ruler! These rulers now represent the distances in grid units that the light traveled from the plane to each satellite.
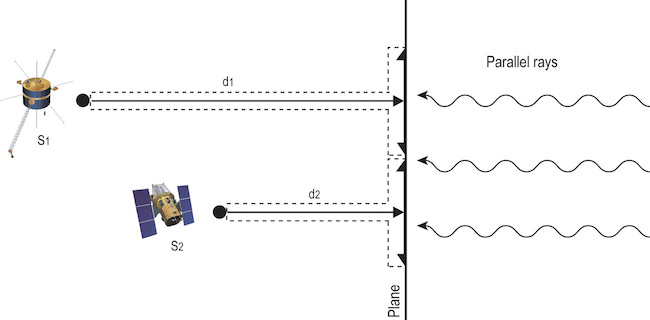
Now, put the bottom end (the end opposite the long arrow's head) of each light ruler on the graph so that it is on the position of the satellite it represents. Rotate the rulers around until the short arrows are lined up. It may help to use a straight-edge to line them up. Align them as accurately as you can! The direction that the short arrows point defines the plane, while the long arrows point in the direction to the gamma-ray burst.
Once the rulers are aligned, lay down your straight-edge over the short arrows on the light rulers and draw the line representing the plane. If you want, you can leave gaps where the light rulers are. Remove the light rulers, then fill in the gaps using your straight-edge. Make sure you extend the line as far as you can on the graph paper.
Once you have drawn the line, place the light rulers back on their satellites. Now, starting where you left off before, continue to rotate the light rulers around. Is there another position where the two light rulers align as they did before?
When you find another position like the first one, mark it with your straight-edge as you did before. Extend it as far as you can on the graph paper. This line represents a second possible plane, and therefore a second possible direction to the gamma-ray burst. This is a problem: there are two possible solutions to the direction to the gamma-ray burst! We need a tie-breaker, and for that we need to use a third spacecraft.
Step 3: Adding a Third Satellite
We'll use data from the Ulysses spacecraft and proceed as before with the other two. Use the table below to add Ulysses to your graph. Call it S3.
| Satellite Name | Satellite designation | Coordinates (light minutes) |
| Ulysses | S3 | (-10, 10) |
On May 6, 2000, at 9:42:24 UT, satellite S3 detected the same gamma-ray burst as the other two spacecraft did.
Question 6: How long did it take (in minutes and seconds) for the light to reach satellite S3 after it passed by the parallel plane?
Question 7: Convert to decimal minutes (for example, 1 minute and 30 seconds = 1.5 minutes).
Question 8: What distance (in meters) did the light travel to reach the satellite S3 after it passed the plane?
Question 9: How many light minutes distance did the rays travel after they passed the plane?
Question 10: Use the grid scale to convert this distance to centimeters on the graph.
Step 4: Finding the Direction to the Gamma Ray Burst
As you did before, measure, mark, and cut the third light ruler so that its length represents the light travel distance you found in Question 9. Place the bottom of the light ruler on S3, and rotate it around. You should find that the short arrow aligns with only one of the two lines representing possible planes. Label this line as the correct one. That's the plane that is perpendicular to the direction of the GRB.
However, simply knowing the direction isn't good enough; astronomers will want a number representing the angle from the Earth to the gamma-ray burst. You'll need to measure that angle with a protractor. First, find a perpendiculat line that connects the Earth to the plane. Hint: you have already done this with the light ruler! Draw the line.
Question 11: Assuming the x-axis represents 0°r;, and that the angle increases counter-clockwise, measure the angle from the x-axis to the line connecting the Earth and the plane.
Question 12; Reflection: Explain at least two properties of gamma-ray light (for example, how is it different or similar to visible light?).
Congratulations! Now astronomers back on Earth will know where to point their telescopes to follow up on this burst.
Math extension (optional, for geometry and trigonometry students):
In reality, astronomers won't draw the lines and use a protractor. They use trigonometry to determine the angle. To determine the angle, first make sure the line representing the plane is extended so that it intersects the x-axis of your graph. You now have a right triangle with one side whose length you have already calculated. From this you can find the angle from the Earth to the GRB as measured from the x-axis.
Question 13: Write down trigonometric formulae that show how to compute the value of the angle using sines, cosines, and tangents of the lengths of the different sides of the triangle.
Question 14: Using each of those trigonometric formulae, calculate the value of the angle from the Earth to the gamma-ray burst in degrees.
Question 15: Average the three angles to get a predicted direction to the gamma-ray burst.
Question 16: Compare this value to what you measured in question 11, and comment on the difference if there is any.
Question 17: Write down a general equation for the angle from the Earth to the satellites S1 and S3 with respect to the x-axis (Satellite 2, Swift, is orbiting the Earth, so we have assumed it is at Earth's position.
Question 18: Calculate the value of the two angles.



