Gamma-Ray Bursts - When You're Hot You're Hot...Unless You're Not!
5. When You're Hot, You're Hot...Unless You're Not!
Most of the information we have about the Universe was obtained by detecting the radiation the object or event emits. This radiation may be the result of a thermal, nuclear, or electromagnetic process. In this activity, we concentrate on the thermal process, that is, things emit radiation because they are hot and trying to cool off.
Let us think about what can happen when electromagnetic radiation is incident upon an object. Three things can happen to the radiation: it can be reflected, transmitted, or absorbed. Unless the object is transparent, the transmitted radiation is negligible compared to what is absorbed or reflected, so we’ll ignore it here. The energy of the absorbed radiation serves to heat the object, which causes the object to begin to radiate away this heat at some point. Thus, it can be said that an opaque object such as a planet can be seen both by the radiation it reflects and by the reradiated energy that it had previously absorbed.
How the total energy of the reflected radiation from an object is distributed in energy (or wavelength) is determined by the absorbing properties of the object. If for example, white light is shone upon the object and it preferentially absorbs longer (red) wavelengths, the object will appear to be blue. Many complicated factors determine the nature of the reradiated energy from the object, but the most important is the temperature, especially if the object is in equilibrium (i.e., it is absorbing and radiating at the same rate so the temperature is constant). To avoid all these complications, scientists often refer to something called a "blackbody". This is a hypothetical, idealized body (or object) that absorbs all of the electromagnetic radiation incident upon it. Its temperature then depends only on the amount of energy striking it and the spectral distribution of the energy it reemits can be exactly calculated by the radiation laws of physics. Here are the main three laws:
Planck’s Radiation Law --- E(l,T) = (2hc2/>l5) ( 1/(ehc/lkT - 1)) gives the radiant energy E emitted per second at any wavelength l by 1 square centimeter of a blackbody at any temperature T. The energy is in ergs, the temperature is in Kelvin, the wavelength is in centimeters, and the Boltzmann constant k is 1.37x10-16.
Wien’s Law --- lmax = constant/T where the wavelength is in centimeters, the temperature in Kelvin, and the constant is equal to 0.2897.
Stephan-Boltzmann Law --- E(T) = sT4 where E is the total energy emitted per second per square centimeter by a blackbody at temperature T. If T is in Kelvin and E(T) is in ergs/cm2/sec, then s (the Stephan-Boltzmann constant) is equal to 5.672x10-5.
It is important to note that a blackbody, being a perfect absorber and radiator, is not necessarily black! At room temperature, it would radiate in the infrared and would indeed appear to be black (since our eyes cannot see infrared). However, a blackbody with a temperature of thousands of degrees would be very bright indeed. Stars are fair approximations of blackbodies because they are composed of hot gases that are very opaque. Because of the high opacity of the gases, the light from a star closely resembles that of a perfect radiator. It is not a perfect fit on the small scale features, but it is a good approximation of the average of all the star’s photospheric layers.
What does this tell us? It tells us that objects of different temperatures can preferentially emit most of their radiation in a different part of the electromagnetic spectrum. In other words, if you want to best see an object of a certain temperature, there is a preferred part of the EM spectrum in which to do the observation!
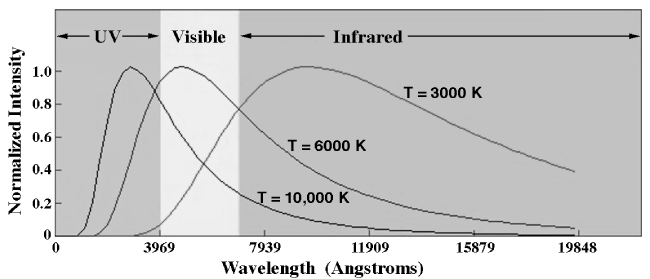
Consider the set of blackbody curves shown below.
You will notice that for each different temperature (3000 K, 6000 K, and 10,000 K), there is a peak in the amount of power measured at a given wavelength. This peak shifts into different parts of the EM spectrum, depending on the temperature.
• Calculate how hot you would have to be to emit thermal blackbody radiation which peaks in gamma-rays (take 10-12 meters as your wavelength of interest).
______________________________________________________
• Compare this value to the hottest things you can think of. For instance, you might consider the center of the Sun.
______________________________________________________
• What conclusion might you be led to concerning the gamma-rays from a gamma-ray burst being thermal in nature?
______________________________________________________