Superstrings

For the last 20 years of his life, Albert Einstein was something of an oddity in the physics community, like a beloved eccentric uncle whose favorite subject of conversation draws embarrassed looks around the table. While quantum theory, the theory of the infinitesimally small, was being tested with accuracy never attained before, he refused to accept that it was the ultimate theory. For the last years of his life, he worked on a way to reconcile his own theory of gravitation and the quantum description of the world. He didn't succeed and died without seeing his dearest dream realized.
More than 40 years later, Einstein is almost vindicated: The long lasting problem of incompatibility between general relativity and quantum mechanics seems to be on its way to a resolution. The solution may be difficult to grasp. If the handful of physicists involved in what are called "superstring theories" (or string, for short) are correct, we live in a world weirder than you can probably imagine.
It's a world of 10 dimensions, with some curled up at a microscopic level and some "big" dimensions that we perceive as "real." A world where the distinction between space and time is spurious (as taught by general relativity). A world where, in fact, the very notion of space and time is bound to disappear. In the words of Brian Greene, a professor at Columbia University and author of a book on the subject,"if string theory is correct, the fabric of our universe has properties that would have dazzled even Einstein."
In string theory, there are no elementary particles (like electrons or quarks), but pieces of vibrating strings. Each vibration mode corresponds to a different particle and determines its charge and its mass. In the current understanding of the theory, those strings are not "made of" anything: they are the fundamental constituent of matter. The consequences of replacing point-like particles by vibrating microscopic strings are enormous. The only consistent framework to describe those strings implies a 10- or even conceivably an 11-dimension world in which 6 or 7 dimensions are curled up. Those extra dimensions are the ones which determine the properties of the world we live in. The larger dimensions are what we perceive as the ordinary space and time.
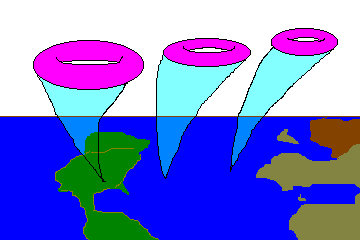
In superstring theory's 10-dimensional spacetime, we still observe only a 4-dimensional spacetime. Somehow we need to link the two if superstrings are to describe our universe. To do this we curl up the extra 6 dimensions into a small compact space. If the size of the compact space is of order the string scale (10-33 cm) we wouldn't be able to detect the presence of these extra dimensions directly - they're just too small. The end result is that we get back to our familiar (3+1)-dimensional world, but there is a tiny "ball" of 6-dimensional space associated with every point in our 4-dimensional universe. This is shown in an extremely schematic way in the illustration to the right.
As a "unified" theory, string theory attempts to explain all four forces observed in nature. And indeed, one of the solutions of the string equations is a force that looks like gravity. It is a testimony to the power and the beauty of string theory that physicists would rather give up the very notion of space and time-- and admit a 10-dimension world--than question the path on which the quest for a unified theory has led them.
String theory could successfully account for gravity and predict super-symmetric particles. But until a couple of years ago it had little connection with puzzles in physics. There were no results or concrete predictions to show off. It could have been nothing more than a beautiful mathematical construction.
Things changed in 1996. Andrew Strominger, then at the Institute for Theoretical Physics in Santa Barbara, and Cumrun Vafa from Harvard University, used string theory to "construct" a certain type of black hole, much the same way one can "construct" a hydrogen atom by jotting down the equations, derived from quantum mechanics, that describe an electron bound to a proton.
Strominger and Vafa confirmed a result derived by Jacob Bekenstein and Stephen Hawking back in the late 1970's. Bekenstein and Hawking found that the amount of disorder (or "entropy") in a special kind of black hole was very large. This was a surprising result, since no one could understand (and nor did the computations give any insight) how an object as simple as a black hole (which can be characterized simply by its mass and its spin) could have such a large amount of disorder within it.
As a result of building this special black hole using string theory, Strominger and Vafa were able to obtain the correct value for the disorder predicted by Bekenstein and Hawking. This result electrified the physics community! For the first time, a result derived with "classical physics" could be obtained from string theory. Even though the black holes for which the result was derived have very little in common with the black holes which are believed to sit in the middle of galaxies, this new computation illustrated the connection between strings and gravity. In addition, the computation provides insight into the physical reasons for the answer.
No one knows yet if string theory is the ultimate theory--the theory of everything, if there is such a thing. But the theory's incredible elegance and potential make it a strong front-runner to further explain the inner workings of the universe well into the next century. In the words of Edward Witten, a pioneer and one of its leaders: "String theory is a part of twenty-first century physics that fell by chance into the twentieth century."
For more information see:


