More on Brightness as a Function of Distance
The intensity or brightness of light as a function of the distance from the light source follows an inverse square relationship. Suppose you were to use a light meter to measure an initial intensity Ii, or brightness, a distance r from a light source. Suppose that some time later the brightness of the light is either greater or lesser; if the intensity diminished you would know that the source was moving away from you and if it became brighter you would know that the source was moving towards you (assuming the light source itself remained the same).
This relationship can be illustrated by the diagram below, which shows the apparent brightness of a source with luminosity L0 at distances r, 2r, 3r, etc. Notice that as the distance increases, the light must spread out over a larger surface and the surface brightness decreases in accordance with a "one over r squared" relationship. The decrease goes as r squared because the area over which the light is spread is proportional to the distance squared.
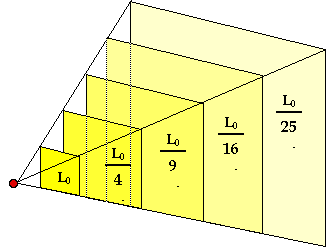
If M31 is moving with respect to the Earth, you should be able to see a change in its apparent brightness if you take two measurements at different times. Measuring this change would allow you to calculate its speed.
See a mathematical derivation of the intensity and distance relationship
See another example of a 1/r2 law
Quiz your knowledge of 1/r2 laws
Return to solving this using the intensity versus distance relationship